Vectors and Probability
-
Some questions here. Would like you guys to help me check my answers. Thanks.(:
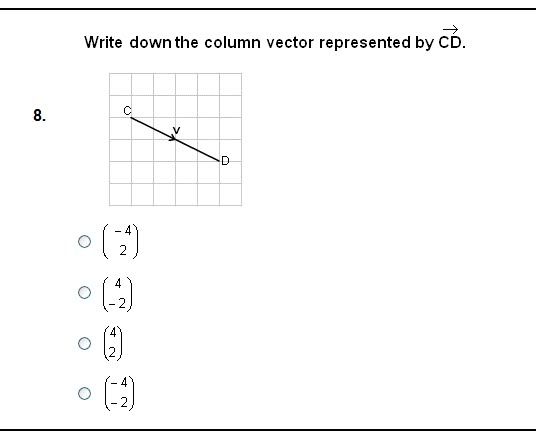
My answer is the 2nd option.(not sure if this's right) But I chose a point of origin 2 squares below popint C. The "y-coordinate" moves down by 2 units the the x-axis needs to shift 4 units to point D. That's how I derived my answer. Is this method valid?

For this I got 10%.
Next comes the most "mind-boggling" question I came across in this assignment. HAHA. Not tough actually, just that I don't know how to present my answers though I got the correct choice.
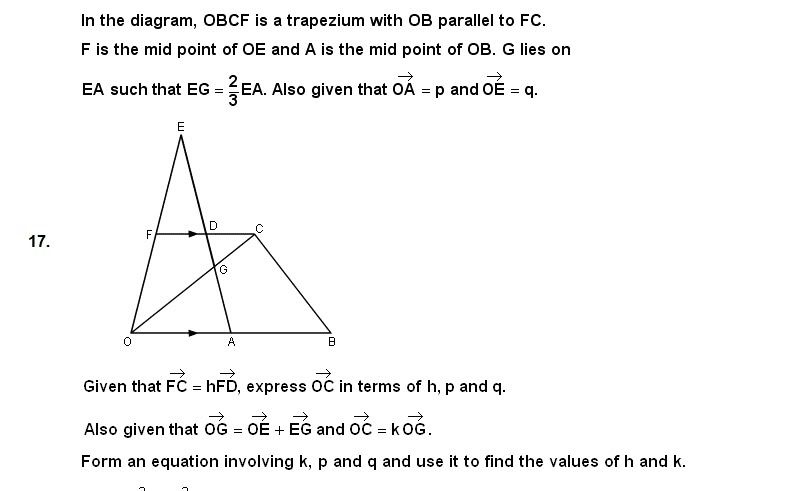
Just need help with presenting the "express vector OC in terms of h, p and q" part
I got h = 2 and k = 3/2.
No problem finding value of k. But for value of h, I actually used the A maths method of intercept theorem to confirm my answer. But I don't really know how to present my answer for the 1st part.
Once again, thanks
-
your answers to the first 2 questions are correct.
-
Originally posted by bonkysleuth:
Some questions here. Would like you guys to help me check my answers. Thanks.(:

My answer is the 2nd option.(not sure if this's right) But I chose a point of origin 2 squares below popint C. The "y-coordinate" moves down by 2 units the the x-axis needs to shift 4 units to point D. That's how I derived my answer. Is this method valid?

For this I got 10%.
Next comes the most "mind-boggling" question I came across in this assignment. HAHA. Not tough actually, just that I don't know how to present my answers though I got the correct choice.

Just need help with presenting the "express vector OC in terms of h, p and q" part
I got h = 2 and k = 3/2.
No problem finding value of k. But for value of h, I actually used the A maths method of intercept theorem to confirm my answer. But I don't really know how to present my answer for the 1st part.
Once again, thanks
For your 1st question, yes, your method is valid.
For your 2nd question, the answer is correct.
For your 3rd question, the workings are as followed:
OF = 1/2 OE = 1/2q
EA = EO + OA = OA - OE = p - q
ED = 1/2 EA ( Since F is the mid point of OE and FC // OB ) = 1/2 ( p - q )
FD = FE + ED = 1/2 q + 1/2 ( p - q ) = 1/2 p
FC = h FD = 1/2 hp
OC = OF + FC = 1/2 q + 1/2 hp