Vectors: E Maths

• anpanman

  16 Sep 09, 18:45

  

   

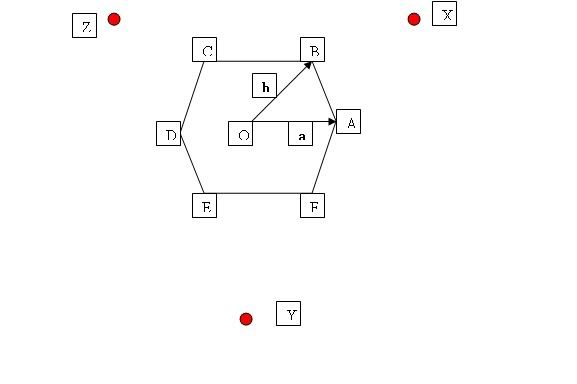
  A regular hexgon ABCDEF, has centre O. Vector OA = a , vector OB = b

  I have given you guys the guiding questions. The actual question is below. Please explain to me how to prove in equilateral triangle. E Maths paper tomorrow, so yea, help is needed urgently.

  Express as simply as possible , in terms of a and/or b,

  Vector DO, AB, and DB

  Explain why |a| = |b| = |b-a|

  The points X,Y and Z are such that

  Vector OX = a + b, Vector OY = a - 2b , and OZ = b - 2a

  Express as simply as possible in terms of a and/or b,

  Vector AX, Vector YX

  Express as simply as possible in terms of a and/or b, the vector XZ

  Show that triangle XYZ is equilateral. (This is the question)

• wee_ws

  16 Sep 09, 23:20

  Hi,

    To show that XYZ is equilateral, we show that all three sides are equal in magnitude.

    Vector XY = -3b, Vector YZ = -3a + 3b = 3(b - a), Vector XZ = -3a.

    Now we consider their lengths, i.e. XY = 3|b|, YZ = 3|b - a|, XZ = 3|a|.

    By the fact that |a| = |b| = |b - a| (because a regular hexagon is formed by six equilateral triangles), the lengths are equal and we are done.

    Thanks and good luck!

  Cheers,
  Wen Shih